

| Deutsche Version |
| Ohm's Law is the linear proportionality between current and voltage that occurs for most conductors of electricity. A graph of voltage against current is a straight line. The gradient is the resistance. Practitioners rarely speak of potential difference, when electrical voltage (drop) is meant. VIR |
Formulas: V = I × R I = V / R R = V / I
The mathematical formulas of Ohm's Law
Ohm's Law can be rewritten in three ways for calculating current, resistance, and voltage.
If a current I should flow through a resistor R, the voltage V can be calculated.
First Version of the (voltage) formula: V = I × R
If there is a voltage V across a resistor R, a current I flows through it. I can be calculated.
Second Version of the (current) formula: I = V / R
If a current I flows through a resistor, and there is a voltage V across the resistor. R can be calculated.
Third Version of the (resistance) formula: R = V / I
All of these variations of the so called "Ohm's Law" are mathematically equal to one another.
| Name | Formula sign | Unit | Symbol |
| voltage | V or E | volt | V |
| current | I | ampere (amp) | A |
| resistance | R | ohm | Ω |
| power | P | watt | W |
|
What is the formula for electrical current? When the current is constant: I = Δ Q / Δ t I is the current in amps (A) Δ Q is the electric charge in coulombs (C), that flows at time duration of Δ t in seconds (s). Voltage V = current I × resistance R Power P = voltage V × current I |
In electrical conductors, in which the current and voltage are proportional
to each other, ohm's law apply: V ~ I or V ⁄ I = const.
Constantan wires or other metal wires held at a constant temperature meet well ohm's law.
"V ⁄ I = R = const." ist not the law of ohm. It is the definition of the resistance.
Thereafter, in every point, even with a bent curve, the resistance value can be calculated.
For many electrical components such as diodes ohm's law does not apply.
"Ohm's Law" has not been invented by Mr. Ohm
"U ⁄ I = R = const." is not the law of Ohm or Ohm's law. It is the definition of the resistance. |
|
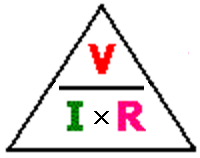
Tip: Ohm's magic triangle The magic V I R triangle can be used to calculate all formulations of ohm's law. Use a finger to hide the value to be calculated. The other two values then show how to do the calculation. |
| Voltage drop calculations - DC / single phase calculation The voltage drop V in volts (V) is equal to the wire current I in amps (A) times twice the wire length L in feet (ft) times the wire resistance per 1000 feet R in ohms (Ω / kft) divided by 1000: Vdrop (V) = Iwire (A) × Rwire (Ω) = Iwire (A) × (2 × L (ft) × Rwire (Ω / kft) / 1000 (ft / kft)) The voltage drop V in volts (V) is equal to the wire current I in amps (A) times twice the wire length L in meters (m) times the wire resistance per 1000 meters R in ohms (Ω / km) divided by 1000: Vdrop (V) = Iwire (A) × Rwire (Ω) = Iwire (A) × (2 × L (m) × Rwire (Ω / km) / 1000 (m / km)) |
If the unit of power P = I × V and of voltage V = I · R is needed,
look for ![]() "The Big Power Formulas":
"The Big Power Formulas":
Calculations: power (watt), voltage, current, resistance
Some persons think that Georg Simon Ohm calculated the "specific resistance".
Therefore they think that only the following can be the true ohm's law.
|
Electrical conductivity (conductance) σ (sigma) = 1/ρ
Specific electrical resistance (resistivity) ρ (rho) = 1/σ
| Electrical conductor |
Electrical conductivity Electrical conductance |
Electrical resistivity Specific resistance |
| silver | σ = 62 S·m/mm² | ρ = 0.0161 Ohm∙mm²/m |
| copper | σ = 58 S·m/mm² | ρ = 0.0172 Ohm∙mm²/m |
| gold | σ = 41 S·m/mm² | ρ = 0.0244 Ohm∙mm²/m |
| aluminium | σ = 36 S·m/mm² | ρ = 0.0277 Ohm∙mm²/m |
| constantan | σ = 2.0 S·m/mm² | ρ = 0.5000 Ohm∙mm²/m |
Difference between electrical resistivity and electrical conductivity
| The conductance in siemens is the reciprocal of the resistance in ohms. |
| Simply enter the value to the left or the right side. The calculator works in both directions of the ↔ sign. |
| The value of the electrical conductivity (conductance) and the specific electrical resistance (resistivity) is a temperature dependent material constant. Mostly it is given at 20 or 25°C. |
| For all conductors the specific resistivity changes with the temperature. In a limited temperature range it is approximately linear: such as T0 = 293.15 K = 20°C at which the electrical resistivity ρ (T0) is known. |
Cross-sectional area - cross section - slice plane
Now there is the question:
How can we calculate the cross sectional area (slice plane) A
from the wire diameter d and vice versa?
Calculation of the cross section A (slice plane) from diameter d:
![]()
r = radius of the wire
d = diameter of the wire
Calculation diameter d from cross section A (slice plane):
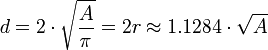
Cross section A of the wire in mm2 inserted in this formula gives the diameter d in mm.
Calculation − Round cables and wires:
• Diameter to cross section and vice versa •
| Ohm's law. V = I × R, where V is the potential across a circuit element, I is the current through it, and R is its resistance. This is not a generally applicable definition of resistance. It is only applicable to ohmic resistors, those whose resistance R is constant over the range of interest and V obeys a strictly linear relation to I. Materials are said to be ohmic when V depends linearly on R. Metals are ohmic so long as one holds their temperature constant. But changing the temperature of a metal changes R slightly. When the current changes rapidly, as when turning on a light, or when using AC sources, slightly non-linear and non-ohmic behavior can be observed. For non-ohmic resistors, R is current-dependent and the definition R = dV/dI is far more useful. This is sometimes called the dynamic resistance. Solid state devices such as thermistors are non-ohmic and non-linear. A thermistor's resistance decreases as it warms up, so its dynamic resistance is negative. Tunnel diodes and some electrochemical processes have a complicated I to V curve with a negative resistance region of operation. The dependence of resistance on current is partly due to the change in the device's temperature with increasing current, but other subtle processes also contribute to change in resistance in solid state devices. |
Calculation: Parallel Resistance (Resistor) Calculator
Color Code Calculator for Resistors
Electric Current, Electric Power, Electricity and Electric Charge
The Formula Wheel - Formulas of Electrical Engineering
In acoustics we use "Ohm's law as acoustic equivalent"
| How electricity works. Ohm's Law clearly explained.  |
| back |
Search Engine |
home |